In essence, rho is the partial derivative of the option value with respect to the interest rate. Carry rho is the partial derivative of the option value with respect to the carry cost. Using the Black-Scholes model in valuation, the carry rho of a call option is given by:
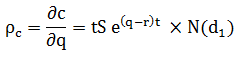
Where: c is the call option’s value, q is the cost of carry, t is time to maturity, and S is the strike price.
The carry rho of a put option is given by:
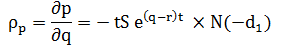
Calculating rho is relatively easy since interest rates have well-established and liquid markets, and thus pricing options is usually a straightforward task using the appropriate section of the yield curve (the yield of a zero-coupon Treasury bond with an identical maturity as the option). Estimating carry rates is a tedious endeavor which uses a non-traded quantity: dividend yields. Future dividends are typically estimated based on the assumption of consistency, i.e., future dividends are assumed to be similar to their historical levels.
Another method for estimating carry rates would be the use of dividend swaps. These swaps do exist but rather occasionally and in a limited range of products. Even when they exist, their market is relatively illiquid.





Comments