A risk measure for options which is computed by relating an option’s theta to its gamma:

Alpha = decay/ gamma

This second-order greek expresses the quality of gamma in terms of the time decay (rent). Therefore, it indicates the quality of the earnings from gamma for one dollar invested in options for one day. A high alpha implies that the premium receiver does not receive sufficient earnings for the costs of the decay. A low alpha reflects the fact that the option trader is getting more for less (more gamma and less theta). Typically, generating higher alphas (for sellers) and lower alphas (for buyers) can be achieved by buying options for low premiums and selling options for high premiums. In this particular meaning, alpha is also known as gamma rent. A more accurate version of alpha is the so-called modified alpha.

Alpha also refers to the sensitivity of an option vega with respect to a change in volatility. It is expressed by

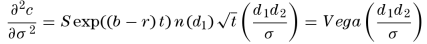
Where: c is a call option, S is the underlying price, b is the carry rate, r is the risk-free interest rate, t is time to expiration, and σ is volatility (standard deviation). Alpha for a put option is given by the same formula.

This option sensitivity is usually expressed as the change in vega for a change of one volatility point (in either direction). For at-the-money options (ATM options), this value is a little bit negative. That is, the vega of an at-the-money option decreases slightly as the volatility increases, and vice versa. However, this effect is insignificant to the extent that the vega of an at-the-money option can presumably be constant with respect to volatility. For out-of-the-money options (OTM options), delta for those with the highest alpha will range between 5 and 10.