Typically, the total amount of interest that a depositor receives is calculated by multiplying the applicable floating rate (say, LIBOR) by the amount of time (deposit tenor), as a proportion of a year, for which the deposit has been active. This amount of time is known as the accrual factor or daycount fraction.
More specifically, accrual factors are computed by dividing the number of days in the deposit period by the number of days in a year. Convention of calculation differs from market to another and across countries. For example, the most popular conventions in use are actual/365 (ACT/365) basis and actual/360 (ACT/360) basis. In all cases, the number of days in the period is measured as the exact number of calendar days within the period (between deposit and withdrawal). However, the number of days in a year varies from one method to another.
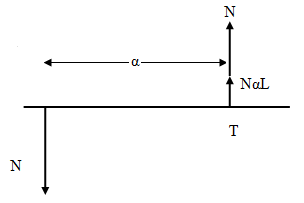
For the actual/360 basis it is assumed to be 360 days; for the actual/365 basis it is assumed to be 365, or 366 in a leap year. Mathematically, if the accrual factor is denoted by α and the corresponding LIBOR by L, a deposit of notional amount N will be paid at maturity along with an interest payment of:
Interest payment = N × α × L
The following figures illustrates how the accrual factor affects the deposit cashflows:





Comments